MIMO Communication Systems via Convex Optimisation and Majorization Theory

Jan 1, 2017

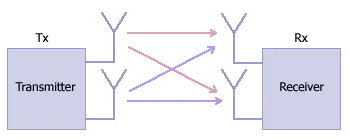
Multiple-input multiple-output (MIMO) channels provide an abstract and unified representation of different physical communication systems, ranging from multi-antenna wireless channels to wireless digital subscriber line systems. They have the key property that several data streams can be simultaneously established. In general, the design of communication systems for MIMO channels is quite involved. The first diffculty lies on how to measure the global performance of such systems given the tradeoff on the performance among the different data streams. Once the problem formulation is defined, the resulting mathematical problem is typically too complicated to be optimally solved as it is a matrix-valued nonconvex optimization problem.

This design problem has been studied for the past three decades (the first papers dating back to the 1970s) motivated initially by cable systems and more recently by wireless multi-antenna systems. The approach was to choose a specific global measure of performance and then to design the system accordingly, either optimally or suboptimally, depending on the difficulty of the problem. We develop a novel unified mathematical framework for the design of point-to-point MIMO transceivers with channel state information at both sides of the link according to an arbitrary cost function as a measure of the system performance. Majorization theory is the underlying mathematical theory on which the framework hinges. It allows the transformation of the originally complicated matrix-valued nonconvex problem into a simple scalar problem. In particular, the additive majorization relation plays a key role in the design of linear MIMO transceivers (i.e., a linear precoder at the transmitter and a linear equalizer at the receiver), whereas the multiplicative majorization relation is the basis for nonlinear decision- feedback MIMO transceivers (i.e., a linear precoder at the transmitter and a decision-feedback equalizer at the receiver).​

Papers